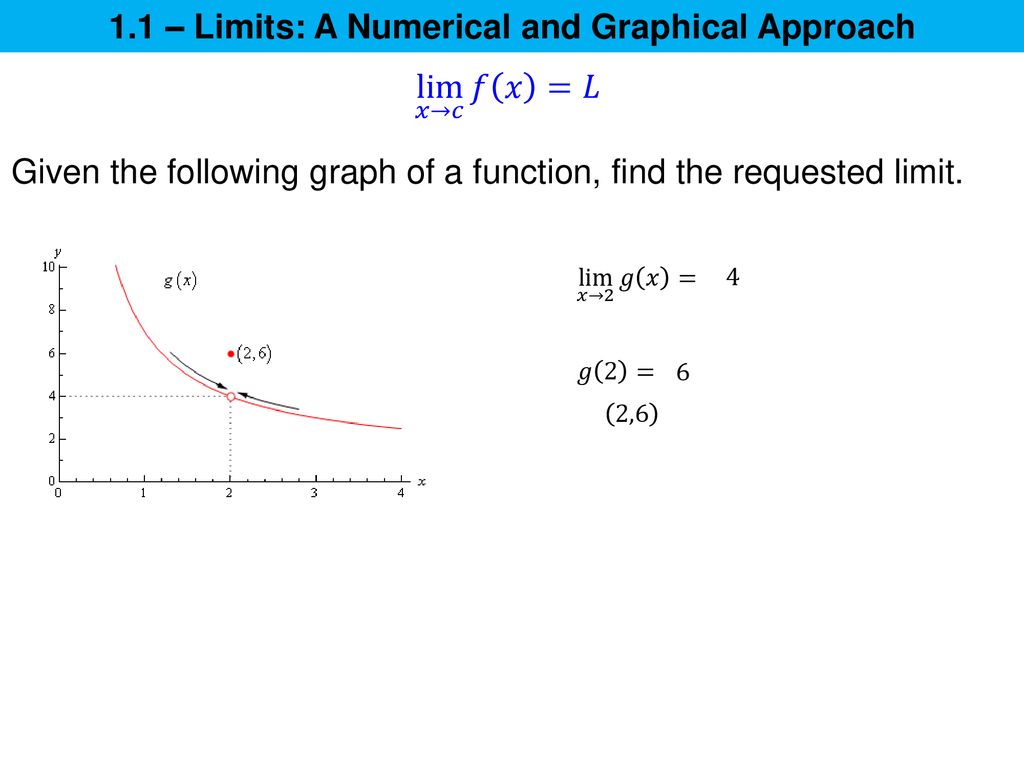
When you're given the graph of a function and your pre-calculus teacher asks you to find the limit, you read values from the graph — something you've been doing ever since you learned what a graph was! If you're looking for a limit from the left, you follow that function from the left-hand side toward the x value in question. Repeat this process from the right to find the right-hand limit. If the y value being approached from the left is the same as the y-value being approached from the right (did the pencils meet?), that y value is the limit. Because the process of graphing a function can be long and complicated, you shouldn't use the graphing approach unless you've been given the graph.
For example, find
in the preceding figure.
1.1 Limits Graphically Calculus Answers
You can see that as the x-value gets closer and closer to –1, the value of the function f(x) approaches 6. And in fact, when x gets to –1, the function's value actually is 6! Technically, though, having f(–1) = 6 isn't required in order to say that the limit is 6; the function could have a hole in it at that point, and the limit would still be 6! See the next example:
Home Calendar Zoom. 1.1 Limits Numerically & Graphically. Complete Hw Notes 1.1 Key. HW 1.1 Key mistake #3b should be -7/3. If ny love for you were an equation it'd be Because it has no limit. 1.1 Limits Graphically Calculus For 1-5, give the value of each statement. Name: If the value does not exist, write ' Practice does not exist' or 'undefined.' F(l) = f(-l) = limf(x) = lim f(x) = i. Explain why direct substitution can not be used to evaluate the limit. Sketch a graph of the function. Use the graph to guess the value of the limit, or explain why it does not exists. Evaluate the function atx— -0.1, 0.01, -0.001, 0.001, 0.01, and 0.1. Use the results to guess the value of the limit, or explain why it does not exist. Use 0.9, 0.99, 0.999, 0.9999, 0.99999 and 1.1, 1.01, 1.001, 1.0001, 1.00001 as your table values. At this point, we see from (Figure) and (Figure) that it may be just as easy, if not easier, to estimate a limit of a function by inspecting its graph as it is to estimate the limit by using a table of functional values.
In the graph, you can see a hole in the function at x = 3, which means that the function is undefined — but that doesn't mean you can't state a limit. If you look at the function's values from the left,
and from the right,
you see that the y value approaches 3. So you say that the limit of the function as x approaches 3 is 3.
You can see that the function has a vertical asymptote at x = –5. From the left, the function approaches negative infinity as it nears x = –5. You can express this mathematically as
From the right, the function approaches infinity as it nears x = –5. You write this situation as
Therefore, the limit doesn't exist at this value, because the left-hand limit is negative infinity but the right-hand limit is infinity.
1.1 Limits Graphicallyap Calculus Frq
For a function to have a limit, the left and right limits must be the same. A function can have a hole in the graph at a particular x value but the limit as x approaches this value can still exist, like
When you hear the word 'limits' what comes to mind?
Jenn, Founder Calcworkshop®, 15+ Years Experience (Licensed & Certified Teacher) Clanree hotel contact.
Speed Limits? Credit Limits? Environmental Limits? Energy Limits? Physical Limits? etc.
As you can see, limits are all around us!
Well, in this lesson we're going to explore the world of Limits as they pertain to calculus.
In fact, the Limits concept distinguishes calculus from all other branches of mathematics, as nicely stated by James Stewart in his book, Calculus: Early Transcendentals, in the sense that we are now interested in how things change over time.
With that being said…
…limits are foundational to understanding Calculus.
The best thing about this cornerstone topic is that it is easy to understand and master!

Phew!
So what is a limit and why does it matter?
Well, to understand what a limit is, we need to look at a graph.
Why?
Because the crux of what we are going to do can be summed up with one question…
'As you get closer and closer to a particular value along the x-axis, what is the y-value getting closer and closer to?'

Math is Fun, uses the phrase 'approaching' whenever they talk about limits, and that exactly what we are doing. We are getting as close to the desired value without actually touching it.
So, together we're going to look at 29 examples!
These examples cover:
- How To Visualize One-Sided And Two-Sided Limits.
- Continuity.
- Discontinuity.
- How To Approach Infinity From Just One Direction.
1.1 Limits Graphicallyap Calculus 2nd Edition
This lesson will give us the framework necessary to tackle limits algebraically and to be able to conceptualize a derivative.
Ahhh, great things to come!
How to Find Limits Graphically – Video
Finding Limits Graphically
Finding Limits Graphically

in the preceding figure.
1.1 Limits Graphically Calculus Answers
You can see that as the x-value gets closer and closer to –1, the value of the function f(x) approaches 6. And in fact, when x gets to –1, the function's value actually is 6! Technically, though, having f(–1) = 6 isn't required in order to say that the limit is 6; the function could have a hole in it at that point, and the limit would still be 6! See the next example:
Home Calendar Zoom. 1.1 Limits Numerically & Graphically. Complete Hw Notes 1.1 Key. HW 1.1 Key mistake #3b should be -7/3. If ny love for you were an equation it'd be Because it has no limit. 1.1 Limits Graphically Calculus For 1-5, give the value of each statement. Name: If the value does not exist, write ' Practice does not exist' or 'undefined.' F(l) = f(-l) = limf(x) = lim f(x) = i. Explain why direct substitution can not be used to evaluate the limit. Sketch a graph of the function. Use the graph to guess the value of the limit, or explain why it does not exists. Evaluate the function atx— -0.1, 0.01, -0.001, 0.001, 0.01, and 0.1. Use the results to guess the value of the limit, or explain why it does not exist. Use 0.9, 0.99, 0.999, 0.9999, 0.99999 and 1.1, 1.01, 1.001, 1.0001, 1.00001 as your table values. At this point, we see from (Figure) and (Figure) that it may be just as easy, if not easier, to estimate a limit of a function by inspecting its graph as it is to estimate the limit by using a table of functional values.
In the graph, you can see a hole in the function at x = 3, which means that the function is undefined — but that doesn't mean you can't state a limit. If you look at the function's values from the left,
and from the right,
you see that the y value approaches 3. So you say that the limit of the function as x approaches 3 is 3.
You can see that the function has a vertical asymptote at x = –5. From the left, the function approaches negative infinity as it nears x = –5. You can express this mathematically as
From the right, the function approaches infinity as it nears x = –5. You write this situation as
Therefore, the limit doesn't exist at this value, because the left-hand limit is negative infinity but the right-hand limit is infinity.
1.1 Limits Graphicallyap Calculus Frq
For a function to have a limit, the left and right limits must be the same. A function can have a hole in the graph at a particular x value but the limit as x approaches this value can still exist, like
When you hear the word 'limits' what comes to mind?
Jenn, Founder Calcworkshop®, 15+ Years Experience (Licensed & Certified Teacher) Clanree hotel contact.
Speed Limits? Credit Limits? Environmental Limits? Energy Limits? Physical Limits? etc.
As you can see, limits are all around us!
Well, in this lesson we're going to explore the world of Limits as they pertain to calculus.
In fact, the Limits concept distinguishes calculus from all other branches of mathematics, as nicely stated by James Stewart in his book, Calculus: Early Transcendentals, in the sense that we are now interested in how things change over time.
With that being said…
…limits are foundational to understanding Calculus.
The best thing about this cornerstone topic is that it is easy to understand and master!
Phew!
So what is a limit and why does it matter?
Well, to understand what a limit is, we need to look at a graph.
Why?
Because the crux of what we are going to do can be summed up with one question…
'As you get closer and closer to a particular value along the x-axis, what is the y-value getting closer and closer to?'
Math is Fun, uses the phrase 'approaching' whenever they talk about limits, and that exactly what we are doing. We are getting as close to the desired value without actually touching it.
So, together we're going to look at 29 examples!
These examples cover:
- How To Visualize One-Sided And Two-Sided Limits.
- Continuity.
- Discontinuity.
- How To Approach Infinity From Just One Direction.
1.1 Limits Graphicallyap Calculus 2nd Edition
This lesson will give us the framework necessary to tackle limits algebraically and to be able to conceptualize a derivative.
Ahhh, great things to come!
How to Find Limits Graphically – Video
Finding Limits Graphically
Finding Limits Graphically
Get access to all the courses and over 150 HD videos with your subscription
1.1 Limits Graphicallyap Calculus Calculator
Monthly, Half-Yearly, and Yearly Plans Available
1.1 Limits Graphicallyap Calculus 14th Edition
Not yet ready to subscribe? Take Calcworkshop for a spin with our FREE limits course
